The Math Profs
|
We hear it all the time.
"My child's so smart! My child's a genius! He's brilliant! So creative!" And then follows the inevitable... "But I can't teach him anything, because he just won't sit still!" (We use "he" and "him" throughout this post, because this problem overwhelmingly happens to boys - including our own. Our girls were model students for us. They listened attentively to every word. They sat still, and did their work most of the time. We thought we were master teachers. And then our boys came along...)
Now, what should the questions be? Well, it depends on the age of your child. The sky's the limit. Any question with an answer from 1 to 100 will suffice.
Examples: 1) When your child is just learning numbers, point to a number on the chart, and have him pronounce it. If he's correct, have him shoot it. 2) Flash cards are great for this! Just have your kid tell you the correct answer out loud before shooting, because Nerf guns are rather imprecise. 3) Do Flash cards without the cards. Meaning, don't show the card to your child, but instead say it aloud to him. It's a good skill to be able to add or subtract or multiply without seeing the numbers on paper. 4) Do word problems. Your child is more likely to fight through the frustration that comes from word problems, if there's the chance to take a shot afterwards. 5) This isn't math, but it's a bonus. Use it for geography. Put a world map on the wall (or a country map, or a state map, etc.), and have them shoot certain countries or oceans as you call them out. This is surprisingly fun. So much so, that the teacher has even been known to take up arms a time or two. (Those European countries are downright devilish to hit on a world map. Good luck shooting Switzerland with a Nerf gun.) Okay, now some will say "Yeah, those sound fine, but it's much more efficient to just have them say the answers out loud to me, or write them on paper. We can go much quicker that way." Guess what? They're right! And if your kid is able to sit still and concentrate well enough for your liking, then by all means do what's most efficient. Our girls did great in that regimen. And we intend to get our boys to that point, as well. But at this stage for our boys, well, it just ain't happenin'! And we're gonna do what works. Even if it's not the most efficient technique. Oh, and even if your child doesn't need "Shoot-the-Number" to stay on task, it's kinda fun to offer it anyway on special occasions. Our daughters don't need it, but they're not averse to pulling the trigger, by any means! Just be warned. A stray projectile sometimes (often?) manages to strike the teacher. By accident, of course.
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
This post may contain affiliate links for which we will earn compensation should you choose to make a purchase. We are disclosing this information in accordance with the Federal Trade Commission’s 16 CFR Part 255, Guides Concerning the Use of Endorsements and Testimonials in Advertising. Thank you for your support of The Math Profs.
1 Comment
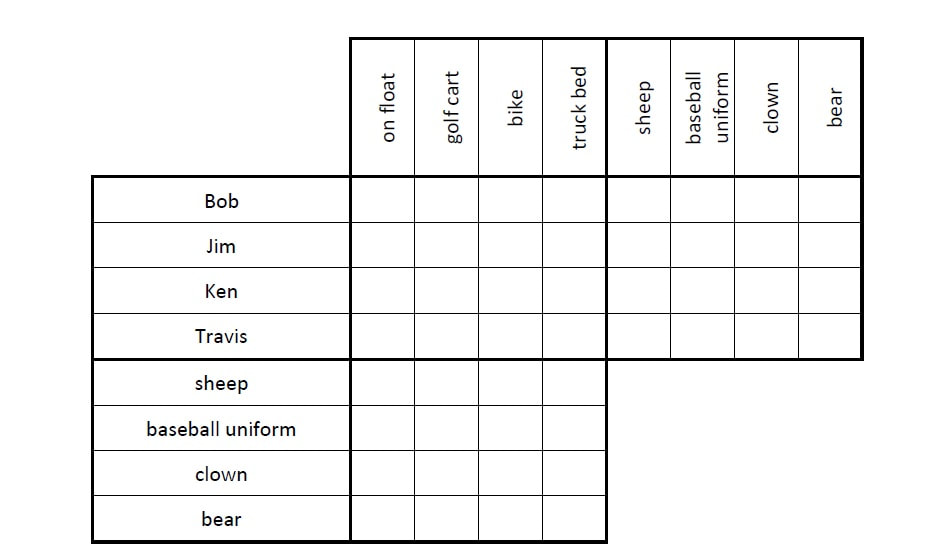
Four people (Bob, Jim, Ken, and Travis) are participating in a parade. They are each riding a different vehicle (on a float, in a golf cart, on a bike, or in a truck bed), and wearing a different costume (sheep, baseball uniform, clown, or bear). Match each person with the appropriate vehicle and costume.
Full tutorial found here.
This puzzle was taken directly from Book 3 of our "Grids For Kids" logic puzzle series. If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
Benefits
If your kids like math, it's pretty easy to teach it to them. Self-motivation makes everything run smoothly.
But what if your kids don't like math? Or what if they're so young, they don't even know what math is? How do you build a foundation and instill concepts? Do you just sit 'em down at a table, hand 'em a hundred 4-digit multiplication problems, and tell 'em they're gonna do 'em till they like 'em? Yes, we've tried it. No, it doesn't work. Instead, we've found the best way to teach math is... to teach it without teaching it.
Confused?
Well, let me say it like this. If you can convey certain concepts without having to resort to chair, desk, and chalkboard, then so much the better! Learning is learning, either way. And we've found there's a greater chance of mutual enjoyment (and retention!) if the teaching occurs unconsciously. This is where games come in. Board games, card games, dice games, you name it. Games are fun, and games can teach. (I'm excluding video games because...well...I just am.) Now, whenever I mention "games" and "teaching kids math", someone always says, "Oh, you're talking about Chess, aren't you?" No, I'm not talking about Chess. I don't know why everyone always associates Math with Chess. (Is it because nerds like math, and nerds like Chess, so therefore Math and Chess must be...the same?) Chess is a wonderful game, don't get me wrong. It can help build concentration, encourage strategic thinking, develop visualization, logic, etc. But Chess is not what I would use to build numeracy.
As a child grows older, subtraction comes into play subconsciously, because that helps determine interval widths for placement. I, myself, use a little probability, together with memory of what cards have already been played.
But without a doubt, the primary educational value of this game is ordering. After several days of playing RACK-O, ordering numbers is second nature. And did I mention that it's fun? It is! Actually, I'm quite addicted. I could play it every night. Trust me, it's a lot more fun than teaching "number ordering" on a chalkboard. So take our advice, and use games to supplement your math instruction. Your kid will enjoy it, and you will as well. Do you have a favorite math game? If so, leave a comment telling us the name of the game and why you like it. We are always on the lookout for new favorites!!
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
This post may contain affiliate links for which we will earn compensation should you choose to make a purchase. We are disclosing this information in accordance with the Federal Trade Commission’s 16 CFR Part 255, Guides Concerning the Use of Endorsements and Testimonials in Advertising. Thank you for your support of The Math Profs.
1. How many unique license plates are possible? 2. Suppose no license plate is allowed to use the letter "O". How many license plates are possible? 3. Suppose no repetitions are allowed--which means that no letter or number can be used more than once. How many license plates are possible? 4. Suppose no repetitions are allowed, and the letter "M" has to be used. How many license plates are possible? 5. Suppose repetitions ARE allowed, but the sequence 211 is NOT allowed. How many license plates are possible? You will find the solution here. To Ponder On Your Own: Would the answer to number 5 change if the disallowed sequence was 111 instead of 211? If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
 A year from now, David's age will be twice Jane's age. Two years ago, David's age was three times Jane's age. How old are David and Jane right now? You will find the solution here. If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
Now, how does that help us? Well, it doesn't….yet. But if we arrange them a little differently into columns, and add each column first, we have \[ \array{ &1 & +& 2&+& \ldots &+ & 99 & + & 100& \\ +&100&+&99&+& \ldots &+& 2 &+&1&\\ \hline \\ =&101&+&101&+& \ldots &+&101&+&101& } \] Do you see what we did? We just rewrote the numbers so that all of the columns would be equal, and then summed each column. Since we know there are \(100 \) columns, we can now use multiplication (much faster than addition) to see that the above is equal to \(100 \times 101=10,100 \). But we're not done yet. In order to make our columns equal, we had to add our sequence twice. So now, to get our final answer, we need to divide \(10,100\) by \(2\) to get \(5050\). [And just remember, a 6-year-old figured this out! Of course, that 6-year-old was none other than Carl Friedrich Gauss, one of the greatest mathematicians of all time. And we really don't know that he was 6, we just know he was in primary school. But still pretty impressive, wouldn't you say?] Now, if you look closely you'll see that there's nothing special about the number 100 in this trick. Any number will work. And you don't even have to remember the steps we took. Let's just make a formula, and then you'll have everything you need. General Formula Suppose \(N \) is a positive integer, and we want to add the numbers \(1 \) through \(N \), written as \(1+2+ \ldots +(N-1)+N \) As before, let's add them twice and line them up in columns: \[ \array{ &1 & +& 2&+& \ldots &+ & (N-1) & + & N& \\ +&N&+&(N-1)&+& \ldots &+& 2 &+&1&\\ \hline \\ =&(N+1)&+&(N+1)&+& \ldots &+&(N+1)&+&(N+1)& } \] This is equal to \((N+1) \times N \), and since we added everything twice to do our trick, we need to divide by 2. And this gives our formula: \[ 1+2+ \ldots +(N-1)+N= \frac{N \times (N+1)}{2} \]. Go ahead and try it out!! It works every time. And here is some homework for you to try. First, try this one: 1. Calculate \(1+2+ \ldots +37+38 \). Now let's see if you can adapt your thinking to these: 2. Calculate \(2+4+ \ldots +98+100 \). 3. Calculate \(20+21+22+ \ldots +79+80 \). 4. Calculate \( 37+40+43+46+ \ldots +94+97+100\). If you have trouble adapting the formula for those last three, you can always add them twice and line up the columns, right? If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
 Question 1: The correct answer is 4 crayons. If Jeremy picks 2 crayons, they might be the same color. Might. But, then again, they might not. And Jeremy wants a guarantee, so 2 crayons won't cut it. What about 3? Nope. He might end up with only one of each color. Will 4 crayons do it? Why, yes! It will! Even in the worst case scenario, where the first 3 crayons are each a different color, the 4th crayon would have to match one of the first 3 crayons since there are only 3 distinct colors. Question 2: The correct answer is 21 crayons. If Jeremy picks 20 crayons, then it's technically possible for him to have 13 blues, 6 browns, and 1 red. So 20 crayons is still not enough to guarantee at least 2 reds. He would need to grab 21 crayons for the guarantee. Question 3: The correct answer is 35 crayons. If Jeremy picks up 34 crayons, he might have all reds and blues. He would need one more to guarantee he has at least 1 crayon of each color. Question 4: The correct answer is 26 crayons. The first 19 crayons Jeremy grabs could be all blues and browns. In this (unlucky) case, he would have 6 browns and would need to grab 7 more crayons from the box, which now contains only the red crayons. And if you still want more puzzles of a similar type, check out our "How Many Socks?" book, for sale at our store www.themathprofs.com. We'll walk you through the process of solving these types of questions - from questions just like these, slowly building to more general scenarios using variables for the numbers of colors and items.
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
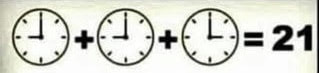
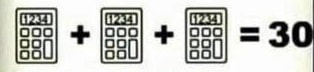
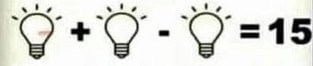
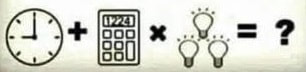
 Jeremy has a box of crayons sitting on a shelf in a very dark room - too dark to distinguish crayon color. Inside this box, there are 21 red crayons, 13 blue crayons, and 6 brown crayons. Now, Jeremy would like to go into the room and grab some crayons out of the box, without taking the whole box. But remember, the room is very dark. And the light doesn't work. And Jeremy's flashlight batteries are dead. And he has no replacement batteries. And he's not allowed to use matches....In other words, Jeremy will have to grab the crayons without seeing what colors they are. Well, Jeremy is a very inquisitive boy, and this quest gets his restless mind working. "How many," he asks himself, "would I have to grab, if I want to make sure I get at least 2 crayons of the same color?" He thinks about this for a minute. "Obviously, I could just grab every crayon in the box, and then I'd know I have some of the same color." He shakes his head at the idea. "But I don't want to grab that many, because then they'll roll off the table, and I'll lose some... and of course little Jenny will eat some..." Jeremy pauses at the door to the dark room. "Then again, I could just grab 2 crayons and hope that they're the same color. But what if they aren't? I really want 2 crayons of the same color. Oh, if only someone could tell me the smallest number that I absolutely have to grab in order to guarantee I have at least 2 crayons of the same color! That's the important thing - I need a GUARANTEE. Surely it doesn't have to be the whole box, does it?" Question 1: What's the smallest number of crayons Jeremy needs to grab in order to guarantee that he has at least 2 crayons of the same color? And if you get that, here are a few more! Question 2: What if Jeremy wants to guarantee that he has at least 2 red crayons? Question 3: What if Jeremy wants to guarantee that he has at least 1 crayon of each color? Question 4: What if Jeremy wants to guarantee that he has more reds than browns? Solution available here. And if you still want more puzzles of a similar type, check out our "How Many Socks?" book, for sale at our store www.themathprofs.com. We'll walk you through the process of solving these types of questions - from questions just like these, slowly building to more general scenarios using variables for the numbers of colors and items. Step 1: Find the value of the clock in Row 4 Note that the clock faces in Row 1 are NOT identical. That's the first mistake people will make, if they're speeding through the puzzle. They'll think the clocks are identical, and thus the sum of the three identical clocks is 21, which gives each clock a value of 7. But since the clocks are not identical, we have to find their value another way. Note that the clock faces are 9, 9, and 3, respectively. Now, what is the sum of \( 9+9+3\) ? Ah-ha!! There's our answer. Each clock has a value equal to the time showing on its face. So the clock in Row 4 is equal to 9. Step 2: Find the value of the calculator in Row 4 If we look at Row 2, we see that this time the objects are identical. Thus, we can say 3 calculators is equal to 30, and therefore 1 calculator is equal to 10. But here we need to be careful, or else we'll make another mistake. To be more precise, we should have said that each of the calculators in Row 2 is equal to 10. You see, the calculator in Row 4 is not the same as the calculators in Row 2. The screen has a different number. So, what do we do? Well, it stands to reason that the value of each calculator is somehow related to its screen number. Does this work for the Row 2 calculators? We found that each Row 2 calculator has a value of 10, and each screen shows 1234. Well, what is the sum of \(1+2+3+4 \) ? Hey! I think we're onto something! If each calculator's value is equal to the sum of the digits showing on its screen, then that calculator in row 4 has a value of \(1+2+2+4=9 \). Step 3: Find the value of the three light bulbs in Row 4. Looking at Row 3, we see that all of the light bulbs are identical. So, on the left-hand side, we're simply starting with 1 light bulb, adding another bulb to it, and then removing the one we just added … which still leaves us with just one light bulb. This means that one light bulb has a value of 15, and therefore the 3 light bulbs in Row 4 are equal to....WAIT!! Did you catch it? I'll admit, I missed it the first time I looked at this problem. The light bulbs in Row 4 are NOT the same as the light bulbs in Row 3. The bulbs in Row 3 each have 5 light rays above each bulb. However, the bulbs in Row 4 each have 4 light rays. What does this mean? Well, it implies that the value of a light bulb somehow depends on the number of light rays above that bulb. We know that each bulb with 5 light rays has a value of 15. To me, this suggests that each light ray contributes a value of 3 to the total bulb value. So, if I want to know the value of a light bulb, I just count up the number of light rays above the bulb and multiply by 3. Since each bulb in Row 4 has 4 light rays, this means that each Row 4 light bulb has a value of \(4 \times 3 = 12\). And therefore, the 3 bulbs in Row 4 are equal to \(3 \times 12 = 36\). (Whew!! Now do you understand why I said this puzzle will test your observational skills, rather than your mathematical abilities?) Step 4: Putting everything together. Okay, substituting for the clock, the calculator, and the 3 light bulbs, Row 4 becomes \(9+10\times36\) Note that the second operation is multiplication, and not addition. (This detail is easily missed by the eyes when glancing from symbol to symbol.) And the Rules for Order of Operations tells us the multiplication is performed first, before the addition. Therefore, Row 4 is given by \(9+360=369\). Afterword: Okay, so 369 is our preferred solution. Now, usually in mathematics we don't speak of a preferred solution. We simply say the solution. But, unfortunately, for a lot of the viral puzzles floating around on social media, the rules are a little....vague. Maybe this helps make puzzles a bit more interesting (we're being generous, here), but it definitely creates some problems. Instead of being able to say "This follows from this, which follows from this, and therefore the answer is this," we are reduced to saying, "I think the best answer is given by this, because I think this is what the puzzle creator intended." Now, I want you to go back to our solution, and look at the assumptions we made. I can see several. For instance, when we noticed that the clock faces in Row 1 were different and also added up to 21, we quickly assumed the puzzle creator intended for clock values to be based on the number shown by the clock face. I think that's a pretty reasonable assumption, don't you? And I think most people would agree. But at the same time, the puzzle creator might have just put different numbers on the faces for variation, and really meant for all clock values to be the same no matter the face value. I doubt it. But it's possible. And that would lead to a different final answer, wouldn't it? But what about the calculator assumption? Once I saw that a calculator screen with 1234 was associated with the value 10, the first thought that occurred to me was "Oh, I just add the screen digits together and that gives the value." But others might have come up with a different scheme. And there really are infinitely many possibilities. I mean, basically you're just looking for a function that takes four input values (the four digits on the screen) and gives one output value. So the function will take the form \(f(x_1, x_2, x_3, x_4)=y \). Now, the only restriction on our creativity in building such a function is that a calculator screen with "1234" on it must have a value of 10. So our function must satisfy \(f(1,2,3,4)=10 \). The function I chose (where I simply summed up the screen digits) could be written as \(f(x_1, x_2, x_3, x_4)=x_1+x_2+x_3+x_4 \). But I can just as easily come up with a different function that still meets our criteria. What about \(g(x_1, x_2, x_3, x_4)=2x_2+2x_3 \)? Does that satisfy our condition? Indeed it does! Check it and you'll see that \(g(1,2,3,4)=2(2)+2(3)=10 \). And that function would yield a different final answer for our puzzle solution, right? In fact, do you realize that if you give me any number in the world, then I can make the puzzle solution equal to that number, simply by creatively choosing the function that evaluates the calculator screens?! And, since the rules are vague, all I would need to do is be willing to argue its defence in an authoritative manner, and I could claim that my solution is the correct solution, and everyone else is wrong - including the puzzle creator, if need be. But rather than go that route, I'd just stick with our preferred solution above, and most folks will agree with you without the need for added argument. Homework: And we can't leave you without some homework!!
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
|
Archives
February 2021
CategoriesAuthorsBrian and Melanie Fulton both earned doctoral degrees in mathematics at Virginia Tech. They formerly taught math at the university level, and now run a hobby farm while accuracy-checking collegiate mathematics texts. They homeschool their four children, frequently employing the aid of chicken, dairy goat, cat, and dog tutors. |





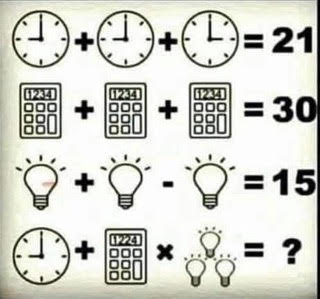
 RSS Feed
RSS Feed
