The Math Profs
|
If you enjoy these puzzles (or if you need help learning to solve them) then our new book Crack the Safe: Level 2 is now available. This new book contains 31 unique puzzles and includes full, detailed solutions.
12 Comments
Our second book in the "Crack The Safe" logic series is now complete, and available for purchase!!! In Level 2, digits might (and might not) be repeated. So, to get your logic juices flowing, here's one of the early (i.e. easier) puzzles in the book. We'll follow with a tougher one later. If you enjoyed these (or if you need help learning to solve them) then our new book series Crack the Safe is now available. This new book contains 31 unique puzzles and includes full, detailed solutions.
We had already started them on some light arithmetic, and I decided it wouldn't be bad to bring in some real-world consumer mathematics. So I started Sales Paper Math Days. Each day that we received a sales paper in the mail from the local grocery store, I sat down with my younger "math students" and we started looking at ads. One would inevitably point at the apples, and quickly ask if he could have one. "No right now," I would answer, "but can you tell me the price of one pound of apples? What about two pounds? Four pounds?" And so on. After going through the whole sales paper, I would give each of them a shopping list, and ask for the total price. Obviously, each list is tailored to the child's age, but I always try to include things like "Two of this item," or "Three pounds of that item." Though I can't claim to have produced savvy shoppers yet, and I still wouldn't trust them to pick out my groceries, they at least have a rough familiarity with going prices. They also see the usefulness (if they're being honest) of arithmetic. And I no longer have to worry about them forking over $78 for a dozen eggs! Activities (based on age) 1) Just read the sales paper for number recognition. 2) Ask easy arithmetic problems that arise while reading the sales paper (for example, "If one box costs this much, how much will two boxes cost?"). 3) Give a shopping list to the child, and have them calculate the total bill. Make sure and include multiple items like "Two of this item" or "Three pounds of that item." 4) Discuss the price per unit for different units (like dollars per pound, or cents per ounce). If the ad allows, have the child compare prices on similar items, and see which item is truly cheaper. If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
Though there are multitudes of interesting tidbits about Newton's life, we'll mention only two: one from his boyhood, and one from his later years. Tidbit #1: Did you know that Newton was at one point only a mediocre student? He was! He had little interest in what was being taught, and was too busy creating sundials and water clocks (he was always a great mechanic). Then one day Newton had a fight with a bully at school, and thrashed him. But beating up the bully wasn't enough for Newton. It galled him that the bully's marks were higher than his, so he decided to devote himself to the school's curriculum. Newton quickly excelled, attracted the teacher's attention, and then never looked back. Who says nothing good comes from a bully?! Tidbit #2: In his later years, Newton was actually entrusted with the integrity of England's currency. Seriously! It was originally supposed to be a symbolic appointment, meant to reward him for raising England's academic prestige with his massive scientific accomplishments. But Newton didn't treat anything lightly, if his name was going to be attached to it. He used his knowledge of chemistry and mathematics to literally hound counterfeiters to death. No joke! He actually had one major counterfeiter hanged, drawn, quartered, and publicly disemboweled! Messing with a nation's currency value and money supply was an attack against every person in the country, and Newton wouldn't have any of it. So here's to Newton. The Man, the Myth, the Legend... ...and the Executioner! If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
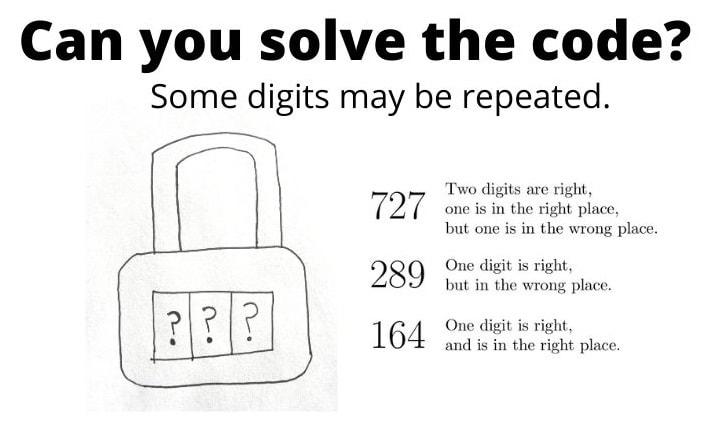
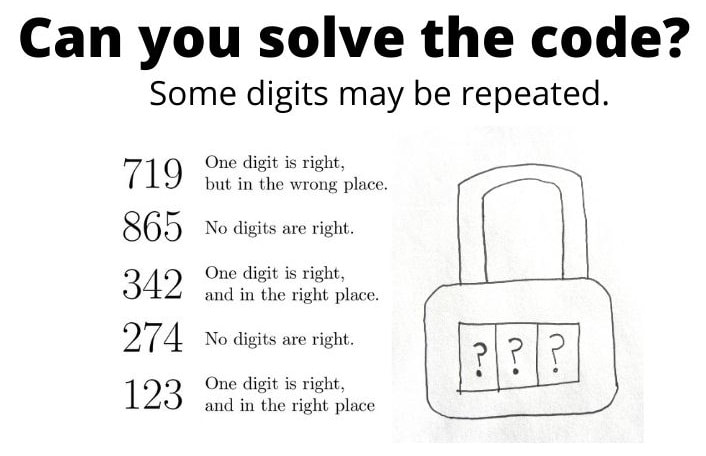
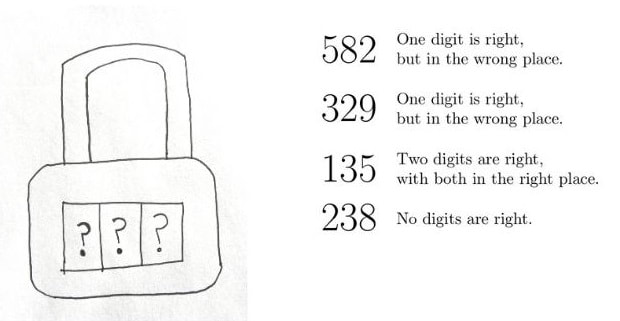
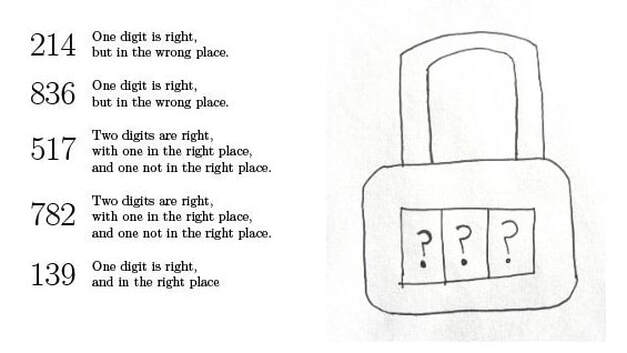
To crack the safe, you need to logically deduce the combinations for the two locks below. Lock One--EasyLock Two--More ChallengingIf you enjoyed these (or if you need help learning to solve them) then our new book series Crack the Safe is now available. This new book contains 30 unique puzzles and includes full, detailed solutions.
The purpose of the Maupertuis expedition was to measure the length of a degree of latitude along a meridian near the North Pole. By comparing their measurements with similar measurements near the equator in South America, Celsius and Maupertuis were able to settle a longstanding debate regarding the shape of the earth. They proved (after a lot of argumentation) that Newton was correct in his theory that the earth was "flattened" at the poles. Today, we also celebrate the achievements of a family friend, Lord Sunflash, who has just been offered a full scholarship to Missouri University of Science and Technology. Congratulations, Lord Sunflash! May your reign be peaceful and profitable, for all your loyal subjects! If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!

The featured Math Game this week involves no arithmetic, no counting, and no calculation. But it is an excellent (and enjoyable) introduction to the concept of plotting points on the Cartesian plane. Granted, the orientation of the vertical axis in the game is inverted from the usual Cartesian y-axis: the index increases, rather than decreases, as one moves downward. And points are plotted in the open squares, rather than on the intersections of the grid lines. But these are small issues, and we've found they cause no problems for children as they transition to the real Cartesian plane.
Variations
Now, if you really want to build familiarity with the actual Cartesian plane, one variation is to use a paper printout of the xy plane. (They're available on TeachersPayTeachers.com for $2.00 or less.) Or, you can make your own xy plane, with coordinate limits of your choice. You can make it as large as you want. And I would recommend you go ahead and center your printout at the origin, so that both positive and negative coordinates are used. It's never too early to introduce the concept of negative numbers. When you're ready to play, simply draw an oval of the appropriate size around the string of points where you want your ship to be. When your opponent scores a "hit", place an X on the point within the oval where the "hit" occurred. To begin with, your children will fire in a somewhat random fashion, naming points as the mood strikes them. But as they mature, you can help them develop a more systematic approach. Teach them to cover the board with a coarse "net" of shots. Then, if the small ships still escape undetected, gradually refine the "net" by subdividing the open sections. When your children are somewhat more sophisticated, start getting creative with the game, and customize your own weapons. I've played the game sometimes where each player has several mines available, of varying size. A mine has an area effect. When using it, you specify the mine's location, and any portion of a ship within the detonation radius of the mine is blasted. Of course, your opponent has to notify you of the mine's effect, and where the "hits" occurred. I've also allowed a certain number of sonar "pings" per player. When a using a sonar "ping", you specify a square region on the board, and your opponent has to notify you of the presence of any ships within such a square. They don't have to give you the coordinates of the ships. They just have to say "Yes, I have a ship somewhere in there", or "Nope, keep searching". And if they have multiple ships in the square, they have to tell you how many ships there are. In summary, Battleship is a wonderful game. Lots of fun, lots of variations. And it's great for teaching the plotting of points in the plane.
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
This post may contain affiliate links for which we will earn compensation should you choose to make a purchase. We are disclosing this information in accordance with the Federal Trade Commission’s 16 CFR Part 255, Guides Concerning the Use of Endorsements and Testimonials in Advertising. Thank you for your support of The Math Profs. Parents, suppose your child approaches you and asks for an allowance, subject to the following conditions:
Should you accept the Proposal? Answer: Absolutely NOT!!! Some reasonably intelligent folks have answered this question by saying, "Well, maybe if you were a millionaire, and had plenty to spare." Nope. Those folks are underestimating the power of exponents. It wouldn't matter if you were the richest person on earth. In fact, it wouldn't matter if you were as wealthy as the top 50 richest people in the world..... combined. Why? Because your total bill at the end of the 8 weeks would be more than $2.8 trillion!!!! To be precise, the actual bill would be $2,814,749,767,106.55. (Mind you, don't forget the 55 cents - wouldn't want to cheat the poor kid, would you?) Pretty hefty allowance, eh? That's the power of exponents. After the first week, you'd be feeling good, thinking you'd made a sweetheart deal. Actually, you might even feel a bit guilty about taking advantage of your kid. The first week's total payment would be the grand sum of 63 cents. But then, at the end of the second week, some question marks might be popping up in your head. After all, the second week's payment would be $40.32. Seems a bit steep for an allowance. And the worst part is, the first day of the third week will earn a paycheck of $40.96. That's just the first day! See, the paycheck for each day is actually one penny more than all the previous paychecks combined. Now do you understand the power of exponents? Okay, so if you understand exponents, how should you counter your kid's payment proposal? I mean, you'd love the extra help around the house, and you'd like to instill appreciation in your child for a well-earned paycheck. But I think you'd agree that $3 trillion is a bit excessive. Well, one possibility is to answer this way: "Johnny (or Jane), I really appreciate your offer, but I'd hate to work you too hard at your age. You know, 'all work and no play' and all that. So let's keep all of your conditions, except one. I want you to have five Sundays a week, and the vacuuming and outside work (and the paychecks) will only be two days a week." Will that be enough to save your financial solvency? Well, the total bill now comes to $655.35. That's a fair amount of money. But remember, the dishes will be done every day for a year. Vacuuming twice a week. Yard work twice a week (and if you have a big yard, think how much a landscaping service would charge). And don't forget the gardening! Still think it's too steep? Then grow a larger garden, and the kid can pay his own salary with the surplus vegetables. Now, if you really want to skin your kid (as punishment for trying to skin you), you can take it one step further. Allow vacuuming and yard work (and paychecks) on only one day a week. That should be enough to get the weekly mowing and weedeating done. Also, you'll get the vacuuming for that day, and maybe a little gardening. Plus, don't forget you'll have all the dishes washed every day of the year. And how much would that set you back? Ha! A grand total of two dollars and fifty-five cents..... for the entire year!!! Now that's a sweetheart deal!! Ah, the power of exponents.
Back in the good old days, when I was teaching Calculus to undergrads, I remember two types of students: those who could calculate, and those who couldn't. Now, certainly there are other skills which are important for the study of higher mathematics. There's creativity, and imagination, both of which feed into visualization and abstraction. But without the ability to calculate, to do simple arithmetic quickly and easily, the other skills are handicapped.
I had students who could grasp difficult concepts, could creatively plan methods of attack on complex problems, or could abstract fundamental truths from seemingly unrelated data. But they couldn't bring those tools to bear in their full strength, because they had a weak foundation in arithmetic. And they were often slow in following my reasoning on the board, because they were still hung up on a simple factoring that occurred two paragraphs earlier ("Dr. Fulton, where did that come from?"). I had students who got Calculus. They truly understood the conceptual picture. But they struggled solving problems, simply because of arithmetic. Why? I don't know exactly. I can only guess that they were allowed to use calculators throughout grade school, and they missed out on the reflexive training for arithmetic. That's a heartbreaking situation, because it's not easily fixable at a later stage. No Calculus student wants to be told to go back and review multiplication tables.
We mentioned one flash card technique that we've used in our "Shoot-the-Number" post, involving Nerf guns. It involved the child saying the answer, and then shooting it on the number chart if he was right. As he improves, make him get 5 in a row before he can take a shot. Then make him get 5 in a row in under 10 seconds. It's like weightlifting. Keep making it harder, tougher, faster. You want the arithmetic to be reflexive.
Another technique we've used is the trampoline. A child gets to keep jumping on the trampoline, as long as they're doing the flash cards and getting them right. Once they've missed, say, three cards, it's time to get off. Choose an activity your kid enjoys, and do likewise. If you have children of different age levels, you can teach multiple kids at once. We just did this yesterday. One child held up a card, a younger child pronounced the numbers on the card (for number recognition), and an older child answered the multiplication problem. Meanwhile, the child holding up the card was also getting a review. Sometimes, it is useful to call out the card to your child, without letting them see it. It's different, and it will slow them down at first. But it's good. It's important to be able to do mathematics without necessarily relying upon optical signals. It builds visualization. There are many, many variations that can be done with flash cards. Be creative. But be consistent. Use them regularly. They're a good, cheap tool in building an arithmetical foundation that will last... ...to Calculus, and beyond!
If you like our puzzles and explanations, please visit our store and check out our problem-solving and logic puzzle books!
This post may contain affiliate links for which we will earn compensation should you choose to make a purchase. We are disclosing this information in accordance with the Federal Trade Commission’s 16 CFR Part 255, Guides Concerning the Use of Endorsements and Testimonials in Advertising. Thank you for your support of The Math Profs. This puzzle was sent to us by a family member. It requires either a little algebra, or else a little "guess-and-check" work.
|
Archives
February 2021
CategoriesAuthorsBrian and Melanie Fulton both earned doctoral degrees in mathematics at Virginia Tech. They formerly taught math at the university level, and now run a hobby farm while accuracy-checking collegiate mathematics texts. They homeschool their four children, frequently employing the aid of chicken, dairy goat, cat, and dog tutors. |







 RSS Feed
RSS Feed
